Posts: 51 Location: Europe
Wed 13 Jan, 2021 10:55 am
Thanks for your answer.
From your answer I gather that I should have expanded my post and point out that I did not post this question at the start of my search, but rather at the dead-end of it. But I did not, because I did not want to steer the answer with the solutions I already have, but hoped for an approach that I did not yet know about, that is easy and convenient to use and in line with the other methods used in the medieval period.
The most common method of dividing a line into 3 equal segments reasonably complicated and involves drawing lines parallel to each other (or at least, that is the common method I know).
I don't know enough about medieval geometry to know if parallel lines were part of their toolbox, but it feels quite different and far less elegant than 'simply' using a compass to work out the dimensions. So I was wondering if there was a more practical/elegant method of coming up with the division into three.
I also found a method (well, two closely related methods) to construct the division into three equal parts using circles, but again, it is quite complicated and as such feels like quite a step outside the elegant compass work that seems to be used to do most of the laying-out of a sword design.
So I was hoping there is a more elegant solution to drawing the two circles inside a the larger one.
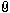 Attachment: 27.29 KB
Attachment: 27.29 KB

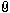 Attachment: 25.7 KB
Attachment: 25.7 KB